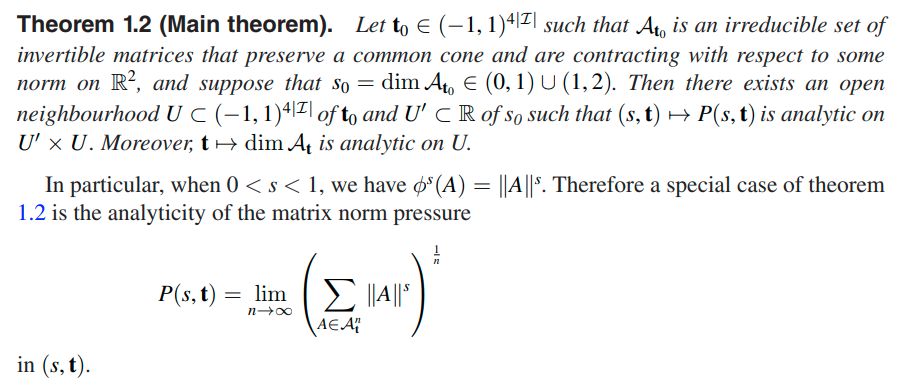
The paper “Analyticity of the affinity dimension for planar iterated function systems with matrices which preserve a cone“, co-authored by Natalia Jurga and Ian Morris, has been published in Nonlinearity (journal link here and arXiv link here). They prove that when the linear parts of the affinities which define the IFS are 2×2 matrices, which strictly preserve a common cone, the sub-additive pressure is locally real analytic as a function of the matrix coefficients of the linear parts of the affinities. Combining this with a recent result of Bárány, Hochman and Rapaport they obtain results concerning the analyticity of the Hausdorff dimension for certain families of planar self-affine sets. A statement of the main theorem is shown below.