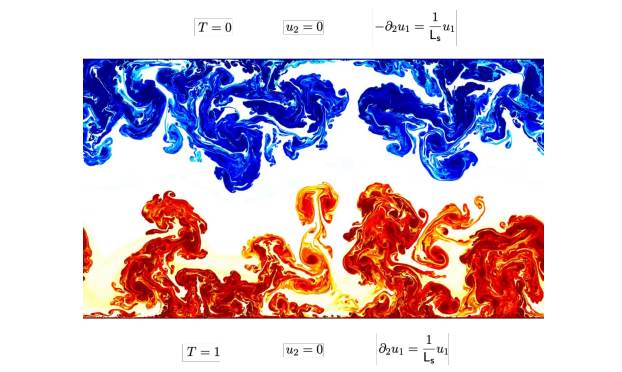
The paper “Bounds on heat flux for Rayleigh–Bénard convection between Navier-slip fixed-temperature boundaries” co-authored by Theodore Drivas (Stonybrook), Huy Nguyen (Brown), and Camilla Nobili (Hamburg/Surrey) has been published in the April issue of the Philosophical Transactions of the Royal Society of London. The paper studies two-dimensional Rayleigh-Bénard convection with Navier-slip, fixed temperature boundary conditions and establishes bounds on the Nusselt number. As the slip-length varies with Rayleigh number Ra, this estimate interpolates between the Whitehead-Doering bound by Ra for free-slip conditions, and the classical Doering-Constantin Ra bound. The published version is here, and the arXiv version is here. The image below shows Figure 1 from the paper.