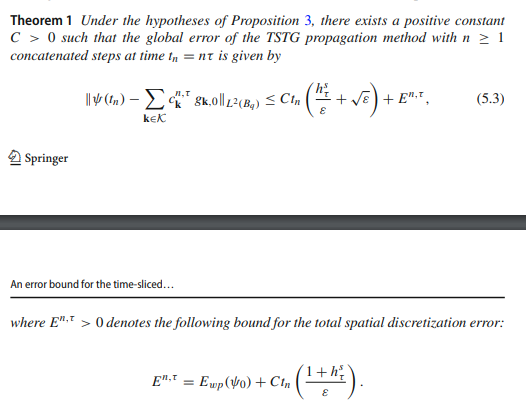
The paper “An error bound for the time-sliced thawed Gaussian propagation method“, co-authored by Paul Bergold and Caroline Lasser (TU München) has been published online and open access this week (22 September) in Numerische Mathematik (publisher’s website here). The paper studies the time-sliced thawed Gaussian propagation method, which was recently proposed for solving the time-dependent Schrödinger equation. A triplet of quadrature-based analysis, synthesis and re-initialization operators are introduced to give a rigorous mathematical formulation of the method. Further, combined error bounds are derived for the discretization of the wave packet transform and the time-propagation of the thawed Gaussian basis functions. Numerical experiments in 1D illustrate the theoretical results. The image below shows a screenshot of the main Theorem of the paper.