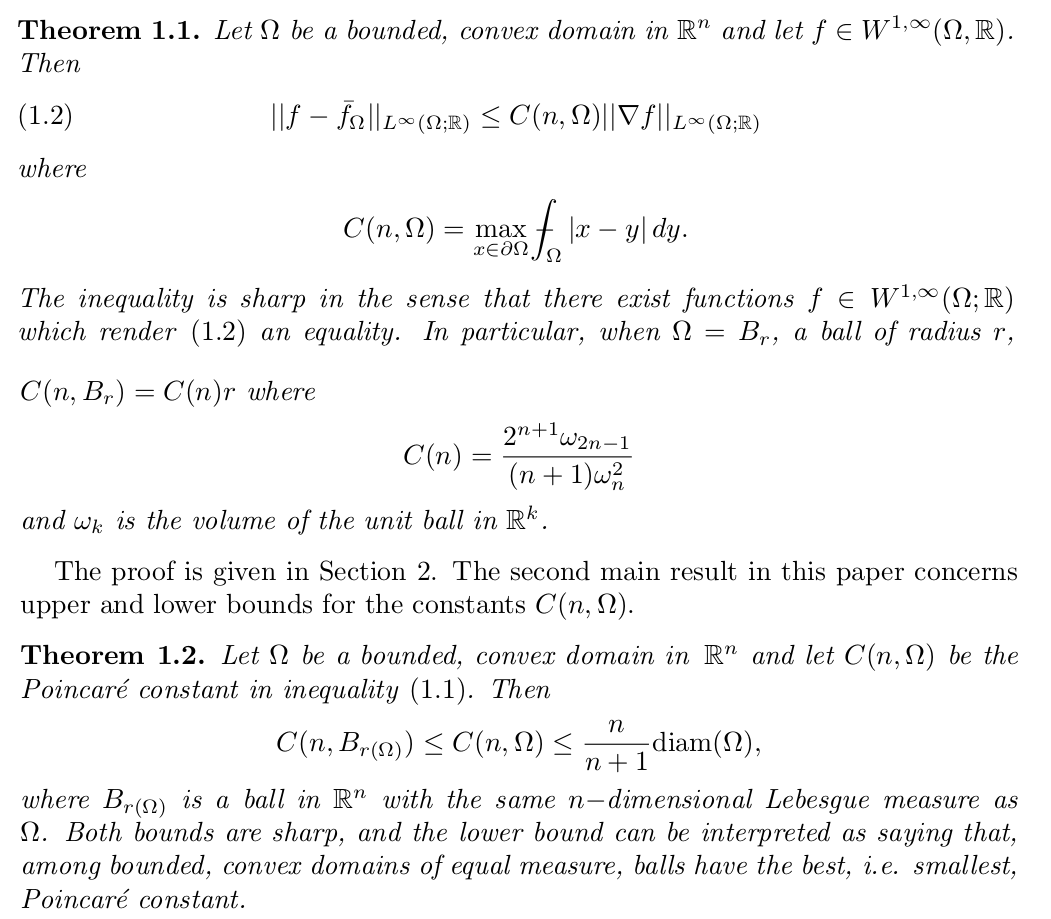
The paper “A sharp Poincaré inequality for functions in W(1,I)“, co-authored by Jonathan Bevan, Jonathan Deane, and Sergey Zelik, has been published in the Proceedings of the American Mathematical Society. The novelty is the proof is in the space of functions W(1,I)=W^{1,\infty}(\Omega,R) and it is sharp. The domain Omega is bounded and convex. A link to the final form preprint and the published version is available (link here). The screenshot below shows the two main Theorems of the paper.