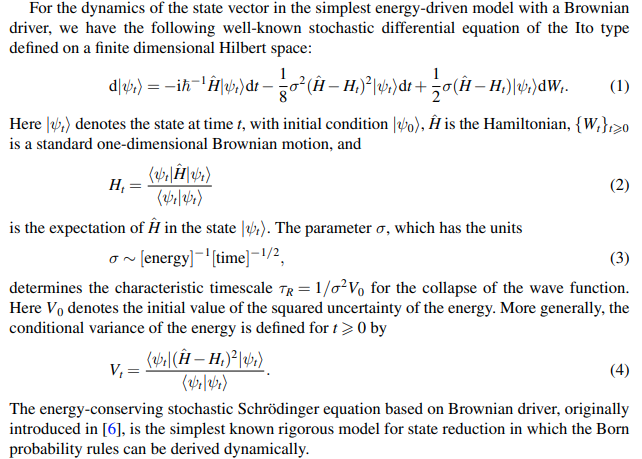
The paper “Lévy models for collapse of the wave function“, co-authored by Dorje Brody and Lane Hughston (Goldsmiths University of London), has been published in the Journal of Physics A. An electronic version of the published paper is available (link here). A brief summary of the paper follows. Stochastic Schrödinger equations characterising the collapse of the wave function are typically driven by Brownian motion. In such models, the decoherence rate is given by energy gaps, which works well for describing small quantum systems, but are problematic in representing macroscopic quantum systems because energy gaps are typically very small. One can ask what happens if the underlying driver is not Brownian motion, but some other Lévy process admitting jumps. In this paper, the dynamics of quantum state reduction driven by an arbitrary Lévy process is worked out in detail. The results show that the associated decoherence rates in the general Lévy case depend not only on energy gaps but also on energy levels. It follows that macroscopic quantum systems will spontaneously collapse into an eigenstate if the state is driven by a Lévy process with jumps. The image below shows one of the key equations in the paper.