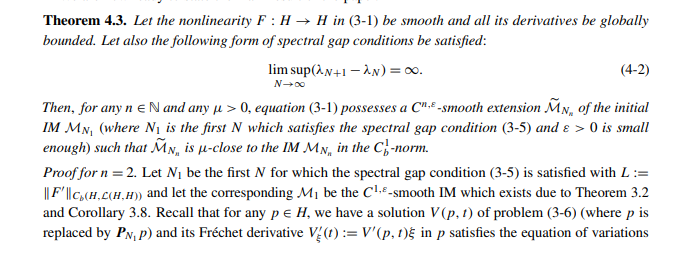
The paper “Smooth extensions for inertial manifolds of semilinear parabolic equations“, co-authored by Anna Kostianko (Zhejiang Normal University) and Sergey Zelik, has been published in Volume 17 of “Analysis and PDEs“. The paper is published open access (link here). The paper is devoted to a comprehensive study of smoothness of inertial manifolds (IMs) for abstract semilinear parabolic problems. The paper shows, under natural assumptions, that the obstacles to the existence of a C^n-smooth inertial manifold (where n is any given number) can be removed by increasing the dimension and by modifying properly the nonlinearity outside of the global attractor. The proof is strongly based on the Whitney extension theorem. A statement of the main Theorem of the paper is shown below.