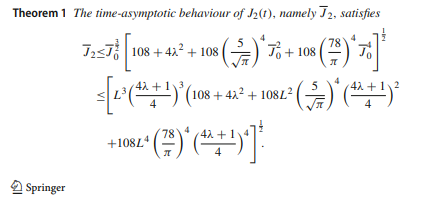
The paper “Explicit estimates on the torus for the sup-norm and the crest factor of solutions of the modified Kuramoto-Sivashinsky equation in one and two space dimensions“, co-authored by Michele Bartuccelli, Jonathan Deane, and Guido Gentile (Roma III, and visiting professor at Surrey), has been published in the Journal of Dynamics and Differential Equations.  A link to the published paper is here. A link to the final-form freely-available manuscript is here.  The photo left show the statement of the key theorem in the two-space dimensional case. J_n is the spatial norm of the n-th derivative and the overline indicates the lim-sup in the limit as t goes to infiinity.