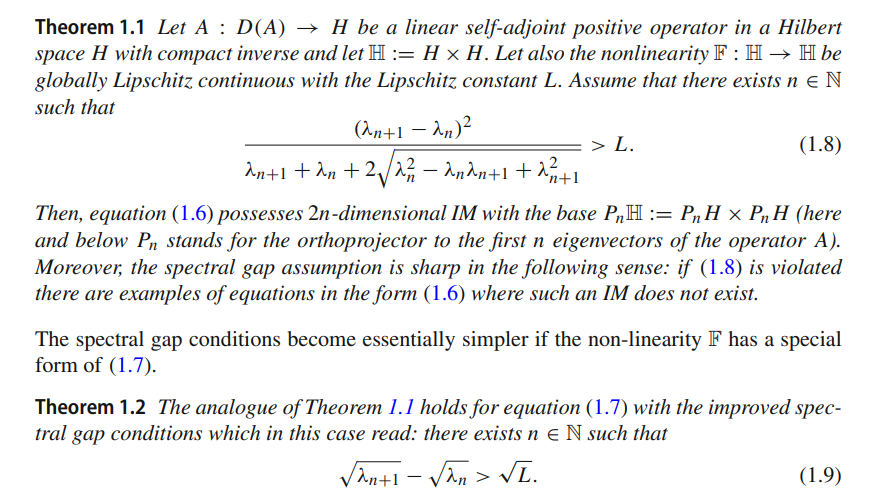
The paper “Kwak transform and inertial manifolds revisited“, co-authored by Anna Kostianko (Imperial College London) and Sergey Zelik, has been published in the Journal of Dynamics and Differential Equations (open access published paper here). The paper gives sharp spectral gap conditions for existence of an inertial manifold (IM) for abstract semilinear parabolic equations with non-selfadjoint leading part. Main attention is paid to the case where this leading part has Jordan cells which appear after applying the so-called Kwak transform. Important examples are the 2D Navier–Stokes equations, and systems of reaction-diffusion-advection equations. The different forms of Kwak transforms and relations between them are also discussed. The image below shows the main theorem from the paper and a special case of it.