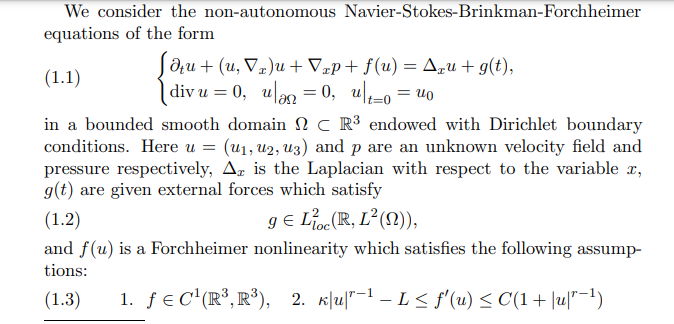
The paper “The non-autonomous Navier-Stokes-Brinkman-Forchheimer equation with Dirichlet boundary conditions: dissipativity, regularity, and attractors“, co-authored by Dominic Stone and Sergey Zelik, has been accepted for publication, open access, in the PRSE. The paper gives a comprehensive study of the 3D Navier-Stokes-Brinkman-Forchheimer equations in a bounded domain endowed with the Dirichlet boundary conditions and non-autonomous external forces. The study includes the questions related with regularity of weak solutions, their dissipativity in higher energy spaces and the existence of the corresponding uniform attractors. The arXiv version is available (link here). The screenshot below shows the key equations in the paper.