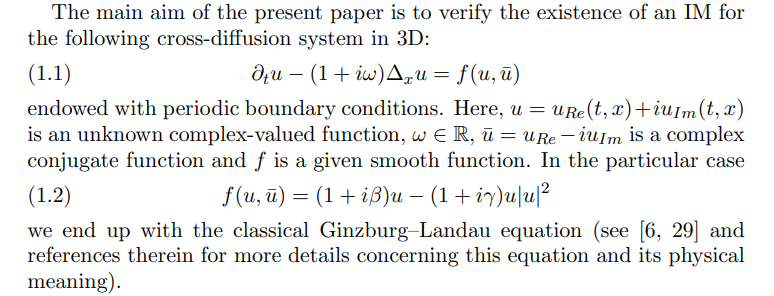
The paper “Inertial manifolds for 3D complex Ginzburg-Landau equations with periodic boundary conditions”, co-authored by Anna Kostianko (Imperial College London), Chunyou Sun (Lanzhou University), and Sergey Zelik, has been accepted for publication in the Indiana University Journal of Mathematics. A link to the arxiv version is here. The paper proves that the complex Ginzburg Landau equation with general smooth nonlinearity, in three space dimensions with periodic boundary conditions, possesses an inertial manifold. The IM is smooth with 1+eps derivatives for some eps > 0. The images below show the version of the CGL considered and a statement of the main theorem.