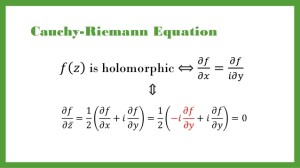
Last week, Sara Pasquetti visited SISSA: Scuola internazionale Superiure di Studi Avanzati in Trieste, Italy.  On Thursday 8th January she gave a talk in the Geometry and Mathematical Physics Seminar on “Holomorphic blocks and compact manifolds“. In recent years a plethora of new exact results for supersymmetric gauge theories formulated on compact manifolds have been obtained via localization, leading to the discovery of new profound correspondences between gauge theories and low dimensional exactly solvable systems such as 2d CFTs and TQFTs. In this talk she presented evidence that partition functions and expectation values of observables of supersymmetric gauge theories on various compact manifolds can be obtained through the gluing of a fundamental set of building blocks, the holomorphic blocks. The decomposition of partition functions into holomorphic blocks corresponds to the geometric decomposition of compact manifolds into standard simpler pieces. Holomorphic blocks have interesting analytic structures and connect to various important quantities in physics and mathematics such as vortex and instanton partition functions, conformal blocks in 2d CFTs and deformations thereof, topological string amplitudes and knot invariants.